Mathematics

California Common Core Mathematics Standards

The California Common Core State Standards: Mathematics reflect the importance of focus, coherence, and rigor as the guiding principles for mathematics instruction and learning. These standards demonstrate a commitment to providing a world-class education for all students that supports college and career readiness and the knowledge and skills necessary to fully participate in the twenty-first-century global economy. The Common Core math standards include two types of standards (seen in the images below):

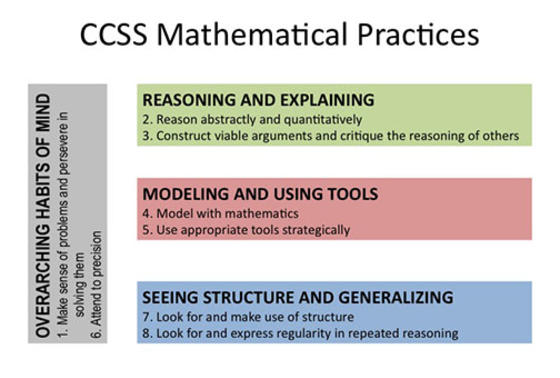
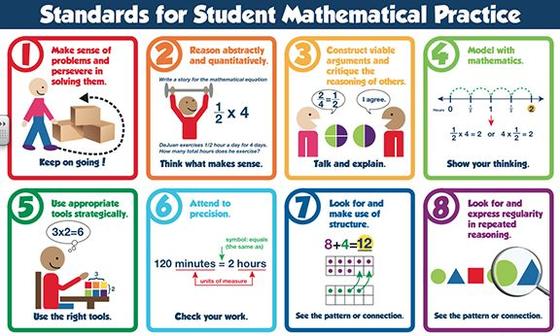
• Eight Mathematical Practice Standards (identical for each grade level) and
• Mathematical Content Standards (different at each grade level).

Together these standards address both “habits of mind” that students should develop to foster mathematical understanding and expertise and skills and knowledge—what students need to know and be able to do. The mathematical content standards were built on progressions of topics across grade levels, informed by both research on children’s cognitive development and by the logical structure of mathematics. The Standards for Mathematical Practice (MP) are the same at each grade level, with the exception of an additional practice standard included in the CA CCSSM for higher mathematics only: MP3.1: Students build proofs by induction and proofs by contradiction. CA This standard may be seen as an extension of Mathematical Practice 3, in which students construct viable arguments and critique the reasoning of others. Ideally, several MP standards will be evident in each lesson as they interact and overlap with each other. The MP standards are not a checklist; they are the basis of mathematics instruction and learning.

CST CA 8th Grade Math vs. CCSS 8th Grade Math

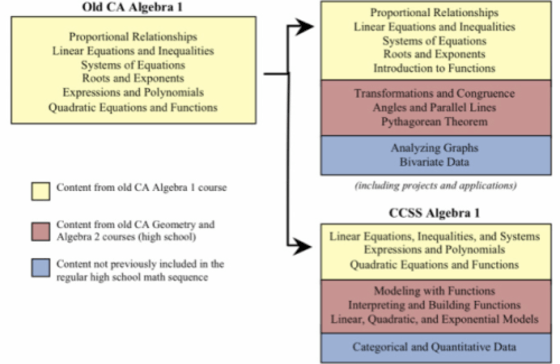
6-8 Mathematics Guides and Resources

• Executive Summary Mathematics Framework
• What Will Your Student Learn: CCCSS Mathematical Standards
• TUSD Mathematical Pathways for Secondary Students (6th -12th Grades)

3 Key Shifts in Mathematics

The following are the key shifts called for by the Common Core in mathematics:

1. Greater focus on fewer topics - Rather than racing to cover many topics in a mile-wide, inch-deep curriculum, the standards significantly narrow and deepen the way time and energy are spent in learning. This means focusing deeply on the major work of each grade as follows:

   • Grade 6: Ratios and proportional relationships, and early algebraic expressions and equations
   • Grade 7: Ratios and proportional relationships, and arithmetic of rational numbers
   • Grade 8: Linear algebra and linear functions

2. Coherence: Linking topics and thinking across grades - The standards are designed around coherent progressions from grade to grade and they reinforce a major topic by utilizing supporting, complementary topics.

3. Rigor: Pursue conceptual understanding, procedural skills and fluency, and application with equal intensity - Rigor refers to deep, authentic command of mathematical concepts, not making math harder or introducing topics at earlier grades. The 3 aspects of rigor are focused upon the following:

• Conceptual understanding: Students must be able to access key concepts from a number of perspectives in order to see math as more than a set of mnemonics or discrete procedures.

• Procedural skills and fluency: Students must practice core functions to have access to more complex concepts and procedures. 

• Application: Correctly applying mathematical knowledge depends on students having a solid conceptual understanding and procedural fluency.